Kristin Brenneman
The Necessity, or Not, of a Definition
chance chan(t)s 
1a: something that happens unpredictably without
discernible human intention or observable cause.
art ärt 4a: the conscious use of skill, taste, and creative imagination
in the production of aesthetic objects; also : works so produced.
While studying these two words and their meanings, one may deny any relationship they have to one another. How could the two, so opposite, merge; how could ``chance" ever produce art? The ``unpredictability" of chance counters the ``consciousness," the ``imagination" of art. But in today's society, many people find it difficult to define art anyway. For example, what some consider offensive, others highly respect as an art form. This constitutes a discrepancy in defining the concept of ART, regardless of what Webster says.
If we stick religiously to the dictionary definition of art, we will never see chance procedures as art. But without such a boundary as this, we can easily find art in a random design. How? As we begin to see that a random process can simulate the same type of qualities human minds produce: patterns of line and color. With the onset of modern (20th century) art in the forms of abstract expressionism, dadaism, and surrealism, humanity has been forced to challenge preconceived ``definitions" of art. Perhaps we must accept as art what we do not consider beautiful or coherent. (Where were those adjectives in the definition?) So, if we categorize these ``artists'" (Pollock, Duchamp, Arp) work, which resemble random outcomes, as art, can't we classify randomizations as art?
In my project, I have not set out to classify or define; rather I have explored the two arenas in conjunction with each other. What role does Chance play in Art? Should the dadaists take credit for their artwork, or should chance itself? How is the computer different from a person's artistic mind, however abstract his designs? As an artist who strives to ``consciously use my skill, taste, and creative imagination" to make art, how do my experimentations with randomized design compare to those who rely upon it for their trademark?
The Dadaists embraced Chance as their avenue to expression in their works of art. They eventually merged random occurrence with conscious creation, attaining a ``balance" between ``art and anti-art" (Richter 60). Jackson Pollock practiced the technique called Action Painting, and also placed value on the complementary forces of the rational and irrational (Rohn 119). He, knowingly some say, thus echoed the Chinese techniques of ages before, in the rhythm of the C'hi (Rohn 42).
Chance in Art. It may seem paradoxical but it is no new concept. The winsome character of a random design enthralls some and gives others cause for scorn. Throughout the past century, many forms of ``abstract" modern art have existed (and even before; it's all relative; at one time Rembrandt was seen as abstract). But still people question the artistic merits of such nonrepresentational works. We must go beyond this question, perhaps satiating it with a ``Yes, these abstractions, randomized as they may be, constitute Art," if we wish to derive any meaning from them. Upon this assumption, we are free to explore the thoughts and methods of premiere modern artists, particularly as they relate to chance.
We can look back to the eighth century and find evidence of randomness in Chinese painting; from their comments we learn that they ``intended the unintentional." Teachings of Taoism led some Chinese artists to believe that chance images could be better explained as symbols of the artist's harmony with the cosmos. Wang Mo, or ``Ink Wang," on the other hand, often got drunk before he splashed paint on a silk scroll, which he then kicked, smeared, scuffed, and sat on to achieve the desired effects (Lachman 501). He finally used a brush at the end of the process, foreshadowing 20th century Dada's characteristic, conscious ``finishing touches" to random designs.
Kinesthetics played a large part in Oriental calligraphy: the artists would suspend their bodies over their scrolls and mobilize their whole body, collecting momentum which they could then drive through their brush to make designs.
This engenders in the stroke a dynamic force as if an electric current had passed through the writer's frame and the brush-handle had been a good conductor; no support 'short-circuits' the passage of the strength into the brush. (Rohn 122)
The word ``support" refers to the controlled actions which result from setting a canvas in front of the body, when only the wrist and arm (after the brain) perform the action of making art. The motion of the whole became very important to these eastern artists of old, and later came to play in the American Jackson Pollock's art of the 20th century.
One look at a painting by this expressionist almost requires the observer to think ``chance." This man stood above his canvas, Oriental style, and moved a paint can through the air, allowing the paint to fall and splatter where it would. He used this technique not as a part of the whole of something more representational, not as one texture among many in a painting, but as the image itself. Splatters on top of each other, in many colors yet usually of the same basic shape and quality, made Pollock's name in the art world. And he said this:
When I am in my painting, I'm not aware of what I'm doing. It is only after a sort of ``get acquainted" period that I see what I have been about. I have no fears about making changes, destroying the image, etc., because the painting has a life of its own. I try to let it come through. It is only when I lose contact with the painting that the result is a mess. Otherwise there is pure harmony, an easy give and take, and the painting comes out well. (Lachman 508)
Regardless of any opinions to the contrary, Pollock never intended ``mess." Of course, all is virtually subjective in art, abstract or not. He said, ``The source of my painting is the unconscious" (Lachman 509). But make no mistake, for ``I can control the flow of paint; there is no accident, just as there is no beginning and no end" (Rohn 114). So, Pollock achieved a state of ``letting go," enough to allow a painting to ``live for itself," not enough to let it control him. As Matthew Rohn states in Visual Dynamics in Jackson Pollock's Abstractions, ``Genuinely random, freely generated strokes of paint would have produced uninteresting, static imagery rather than anything resembling the verve and life of a Pollock" (40). He presents the idea that an abstract artist cannot be compared to a monkey and his random designs because the animal performs with ``no clear visual intent." Though some critics may disagree, he argues that even the human artist's least work will show much more profound imagination than that of a monkey.
Though Pollock remained master of his canvas, certain elements of his drip-methods confounded his complete control, no matter how he believed that there was ``no accident." He couldn't always know how the paint would puddle or how viscous it would be, yet in no way could this in itself set Pollock apart from any artist (Rohn 118). (Even the most realistic painter could encounter a freak in the nature of his medium; this might add a beautiful quality to his artwork and teach him something good, while it might go unnoticed by the observer.) But these factors don't constitute the random quality of Jackson Pollock. Rather, he did vary his method from thoughtful to thoughtless, achieving improvisation in his work. Sometimes he chose where to pour his paint and in what type of stroke; others he relinquished his paint can to the wiles of the irrational, allowing his body to become automated (Rohn 118-20). He interplayed the two dialectical approaches and thus arrived at an expressive ``tension." In essence, Pollock controlled his parameters while welcoming ``the dynamics of spontaneity and flow" (Rohn 42).
Dada: ``the total repudiation of art; the absence of any ulterior motive;" ultimate freedom; the avenue to ``the voice of the 'Unknown'" (Richter 50). Hans Richter, Dadaist and author of Dada: Art and Anti-Art, said this: ``Chance became our trademark. We followed it like a compass." André Masson trickled sand through his fingers onto a canvas as he moved over it in dance-like motions, after first concentrating very hard. ``It is when I have completely switched off my will that my body and my nerves, my subconscious self, know best when and where to let the sand fall" (Richter 55). Marcel Duchamp, inventor of Dadaism in America (while French), experimented with chance especially in his work, 3 stoppages étalon, 1913-14. ``Wind, gravity, and aim" participated as he dropped three threads onto a flat surface and fixed them as random lines (Masheck 39). Thus merged ``precision and unlimited freedom, the actual and the potential" of art, of Duchamp, the forces of thought and of chance. He planned out the problem and invited chance in to work for his end (Masheck 58).
Hans Arp, premier Dadaist, tore up paper, let it fall from his hands to scatter upon a surface, then he glued down the design, having found what he had vainly been striving for in his art of consciousness. He considered such chance patterns to be fate's work, correlating to Dada's premise of the artist trusting in an outside, ``mysterious collaborator" (Richter 51). Arp said this:
The law of chance, which embraces all other laws and is as unfathomable to us as the depths from which all life arises, can only be comprehended by complete surrender to the Unconscious. maintain that whoever submits to the law attains perfect life." (Richter55)
Though more of an abstractionist than a Dadaist, Paul Klee dealt with chance as well, but in more ``abstract" terms:
an active line on a walk, moving freely, without a goal. A walk for a walk's sake. The mobility agent is a point, shifting its position forward. (Brooks 99)
The line has no goal according to Klee, yet as the father of the line he must have had to relinquish its purpose, simply because as a human being he couldn't avoid thinking about the role of the line. It may not have thought, but he thought enough to establish this formula of its unconsciousness. Hence Klee's correlation to the Dadaists-his value of chance in his artwork, yet his status of control over each piece.
Observing the work and listening to the ideas of these artists, we can see how randomness emerged for them as a creative ideal. Perhaps chance itself, in a general sense, cannot constitute art, but these abstractionists' prominent names attest to the fact that they used it to benefit their individual art. They allowed fate or nature to take its course, often instead of using their minds to plan out a pattern. They formulated no rules, because that would defy chance itself, wouldn't it?
stochastic: sequence which combines random components with a selective process so that only certain outcomes of the random are allowed to endure.
While chance runs counter to most people's conceptions of true art (if it is an attempt at portrayal of something real, if it seems to have required skill and creativity), it can greatly improve the symmetrical quality of design. Perhaps this occurred to Arp when he saw the scraps of paper on his floor, in an array he never would have chosen, though captivating nonetheless. Michael Eckersley states,
random graphical arrays, almost without exception, exhibit striking examples of dynamic asymmetry; localized sections of large arrays can be isolated and appreciated as powerful compositions. . .The design process can be viewed as a cybernetic machine in which the rules define the capabilities of the machine, randomness provides the input, and the design product is the output. Once the basics of the machine have been set forth, the creative designer manipulates or redesigns the machine in such a way as to get more satisfactory designs. (Eckersley 78)
These postulates call up two very important aspects of chance in design: its ability to create interesting compositions and its adherence to rules once they are set (programmed) by the human mind. Just like Pollock, the creator controls while chance gives distinct, ultimate life to the image.
Fred Whipple has formulated just such a set of rules, which govern the exhibition of a ``stochastic painting." He prefaces them by saying that creating them (the rules) is an artistic process. In painting or coloring in shapes chosen by randomization, a human hand applies the colors or shading; ``thus, stochastic painting does involve creativity and self- expression, although not of the classical type" (Whipple 81). He has realized that often people interchange the concept of ``irregularity" with ``randomness." To specify the difference, a random design may very well display some regularity, though that goes against our opinion of chance (happening without cause). But the truth is that a regular pattern is just as likely to occur as an irregular one.
The probability of this sequence, HTHTHTHTHT equals that of this one, HTTHTHHHTT, though the second ``looks more random." This illustrates the myth we hold regarding chance, one very difficult to avoid. As humans with the capability of reasoning and discerning, we want to find a pattern in everything. As Stephen Jay Gould says in an article on Streaks,
We see a ``constellation" in the first ten tosses of the coin, though its stars are just as randomly placed as those of the second. Here we get back to the ``art of it all": what the eye finds pleasing is not always planned. A pattern pleases the eye, and if a creator/artist has planned no pattern, the eye will find one. Hence the role of chance in art. We cannot rule it out as having no artistic value, because it deals a dynamic deck of design, including the compelling existence of a pattern here and there, as well as enough ``random-looking" images to cause the mind to wonder.
As for Whipple, he lists nine rules which can be followed to
create a random design. A design of random polygons or
circles, that is (a design of random spots could be attained by
flinging or dropping paint). The rules are listed on an
additional page; his designs are included as well. I have also
made a design following his rules which relate to a randomized
set of numbers. I programmed True Basic (on poster) to find an
infinite number of random points between 0 and 1, display two
at once as 
and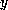 
, and to find 
as 150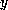, as Whipple describes,
to get an angle from each respective line. I used only two
colors, blue and white, whereas he painted many colors in his
random design. I chose the 2- rather than 3-dimensional
approach, as explained in rule 5.
I experimented with a simpler method of randomizing color for a static set of shapes, small squares as part of a larger square. I was thinking of Mondrian's color block paintings, and I in fact achieved something very similar. I controlled virtually everything but the colors, which I had numbered 1 through 6 though 5, purple, never came up in the first ``Run" screen. I could have colored each block but preferred the effect of a sparser design. This proves one extreme of chance in art: the work is hardly random at all, only the colors detract from its ordered, even quality. The small square I made using Whipple's formula appears to be the other extreme, with nothing close to being uniform or representational.
I utilized two main True Basic programs to create totally
random designs with line and color. The first drew lines in
random directions, which criss-crossed to form connected
polygons, yet usually left one or two stray lines outside the
main form. As the lines appeared, they portrayed an image of a
variable number of white shapes overlapping each other. Once
had been reached, the design retained its shape, and colors
began to fill in individual sections, including the background
(as the large mass was suspended in space). The color was
also programmed to apply times to random areas, and I used
``set color int(rnd*256)" to give the computer a wide palette
from which to ``choose". I ran this program over 30 times and
noticed that within each separate run, the most common area
to fill was the background. Often it would change colors many
times before any one space inside the figure would be colored.
This program did not set the first colors in their respective
spaces. Rather than make more spaces colored than white, the
20 () color ``floods" would most often serve to change the
spaces which were already colored. I printed out only the last
stage of each program's run output, but each process included
different (according to the number color floods ``for n = 1 to
20") ``works of art." I modified this program to draw fewer
lines than I had been working with and only use one color
instead of 256 randomized. I could have controlled the color by
telling it to flood only red, but I happened upon another method
which could keep the chosen color random. I simply switched
the commands ``for n (or i) = 1 to x" and ``set color
int(rnd*256)," and each design became limited to a single
color.
Another control I experimented with in programming was that
of lines extending from the x to y axes. To suspend the shape
in the middle of the screen as I did before, I had used ``plot
lines: rnd, rnd; rnd, rnd;". I simply changed this to ``plot lines:
rnd, 0; 0, rnd;" and the lines all traveled from the left to the
bottom (y to x axis) of the screen. They remained randomized
but they were directed to satisfy a planned shape: a basic
triangle slicing the screen diagonally (if the window was set
at 0, 1, 0, 1). Everything else operated the same, concerning
the color changes. I decreased the window size (0, .7, 0, .7) to
magnify the image and hide the sharp triangle edges. I created
a variation on this program which used one color (still
randomized) at a time to flood the whole screen, and black to
flood random parts of the large triangle and the background.
To do this I added ``set background color rnd" and a variable 
which represented a ``color mix." Before I defined c, I used `` =
1 to 20" to cause the color to change
20 -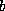 
(no. of times black flooded something) times. Adding
``pause .2" slowed the program enough for me to observe the
changes, which happened quickly.
I found a program which created a checkerboard-type design in the True Basic Reference Manual, using sine and cosine. I replaced those equations with ``rnd," and I started the program with ``randomize," and I discovered the resulting designs to be random and intriguing. I found the work of David Bomberg to look very similar. Though he planned one particular piece out with a sketch of the subject (a man running), this final painting looks like in style, even technique, the computerized picture. The man's form is nearly lost in the maze of blacks and whites-the eye must be trained to look for him and find him. This case brings up the idea of similarity between the mind and a computer, or does it? No matter what Bomberg thought when he designed this and painted it, we know the computer did not take that approach, as it cannot think. The human mind, through the controls of the program, instructed the computer how to execute the design, yet allowed it to randomize the image. We know that with man's intelligence, a computer can perform a growing multitude of unbelievable things. But only from man do the processes originate. We cannot discount Bomberg's talent or creativity simply because a computer can simulate a similar-looking design.
It must all come down to speculations as well as facts, as art is subjective. Just as we have seen it all come down to chance AND thought, or premeditation. Chance holds much power in this world and in the world of art. Because the artists set the pace for that world, we can see that the recognition they have given Chance in this century must deem it worthy of attention, even praise. How interesting that people search for meaning in a design made by a person, when a computer can run an output sheet which could pass for the same artist's work.
I have now added randomization to my repertoire as an artist, but I cannot say I consider these works my own. Though I wrote the programs or followed the rules that made them what they are, and though I controlled chosen factors, I was never responsible for the outcomes. If I didn't think the red should have gone in block 8,3, I still would have put it there so I wouldn't ``break the rules." But here's the fact and the paradox of modern art: I may well have thought all my ``random" designs through and made the decisions myself; there's no way you'll ever really know.
* First create a table of random number pairs.
and 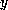on a canvas coordinate
system for the starting point.
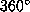, gives a direction from the starting
point; the second, multiplied by a unit distance, say a
centimeter or half an inch, measures a
distance in this direction.
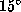, measures an angle turned counterclockwise
from the tip of the previous line.
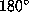. I chose to join the figure at
the end of a line when any projection of a line was pointed towards
the originating side of the
polygon. This rule leads frequently to several lines radiating from
a point, which gives some
sense of three-dimensionality to the final painting. (See Fig. 1.)
This document was generated using the LaTeX2HTML translator Version 0.6.4 (Tues Aug 30 1994) Copyright © 1993, 1994, Nikos Drakos, Computer Based Learning Unit, University of Leeds.
The command line arguments were:
latex2html -split 0 Kristin.tex.
The translation was initiated by J. Laurie Snell on Fri Nov 11 12:51:30 CST 1994