Mathematics 5: Chance Fall 1995
by James E. Baumgartner and Shunhui Zhu
Content
Class 5
Class 6
Class 11
Class 19
Class 20
Class 23
COURSE DESCRIPTION
Welcome to CHANCE!
Mathematics 5: CHANCE is a new, experimental math course. The standard elementary math course develops a body of mathematics in a systematic way and gives some highly simplified real-world examples in the hope of suggesting the importance of the subject. In the course CHANCE, we will choose serious applications of probability and statistics and make these the focus of the course, developing concepts in probability and statistics only to the extent necessary to understand the applications. The goal is to make students more literate in statistics and probability, and to motivate them to continue their study of mathematics.
The journal Chance, started by Springer in l988, is the inspiration for this course. In its brief existence, Chance has attracted some of the leading workers in probability and statistics to write articles on subjects of current interest in a way understandable by a reader with little previous knowledge of probability and statistics. Topics that have been covered in Chance include:
- Air safety
- Scoring streaks and records in sports
- Health risks of electric and magnetic fields
- The effectiveness of aspirin in preventing heart disease
- Statistics, expert witnesses, and the courts
- The undercount problem in the 1990 U.S. Census
- Extraterrestrial communication
- The use of DNA fingerprinting in the courts
- Maintaining quality of manufactured goods in the face of variation
- Randomized clinical trials in assessing risk
- The role of statistics in the study of the AIDS epidemic
- The use of statistics to detect cheating on exams.
Other topics that have been recently discussed in the press and popular journals such as Nature, Science and Scientific American are:
- Paradoxes in probability and statistics
- The work of Kahneman and Tversky on fallacies in
human statistical reasoning
- The stock market and the random walk hypothesis
- Demographic variations in recommended medical treatments
- Informed patient decision making
- Coincidences
- Random and pseudo-random sequences
- The reliability of political polls
- Card shuffling, lotteries, and other gambling issues.
In the course of the term, we will choose six to ten separate topics to discuss with special emphasis on topics currently in the news. We will start by reading a newspaper account of the topic. In most cases this will be the account in the New York Times. We will then study the treatments in journals like Chance, Science, Nature, and Scientific American. These articles will be supplemented by readings on the basic probability and statistics concepts relating to the topic. We will use computer simulations and statistical packages to better illustrate the relevant theoretical concepts.
Organization
The class will differ from traditional math classes in organization as well as in content: The class meetings will emphasize group discussions, rather than the more traditional lecture format. Students will keep journals to record their thoughts and questions, along with their assignments. There will be a major final project .
Scheduled meetings
The class meets from 12:30 to 1:35 on MWF in Room 102 Bradley Hall. Due to the interactive nature of the course, you will be expected to come to class, and engage whole-heartedly in the discussions. The X-hour Tuesday 1:00 to 2:00 will be used for discussion of material in the text, questions about homework, use of the computer, or anything else relating to the course.
Discussion groups
We want to enable everyone to be engaged in discussions while at the same time preserving the unity of the course. From time to time, we will break into discussion groups of 3-6 people.
Every member of each group is expected to take part in the discussion and to make sure that everyone is involved: that everyone is being heard, everyone is listening, that the discussion is not dominated by one or two people, that everyone understands what is going on, and that the group sticks to the subject and really digs in.
After a suitable time, we will ask for reports to the entire class. These will not be formal reports. Rather, we will hold a summary discussion between the teachers and reporters from the individual groups.
Text
The required text for the course is Freedman, Pisani, Purves, and Adhikari, Statistics, 2nd edition and the software package JMP. The text is available at the Dartmouth Bookstore and Wheelock Books, JMP is keyserved.
Journals
Each participant should keep a journal for the course. While assignments given at class meetings go in the journal, the journal is for much more: for independent questions, ideas, and projects. The journal is not for class notes, but for work outside of class. The style of the journal will vary from person to person. Some will find it useful to write short summaries of what went on in class. Any questions suggested by the class work should be in the journal. The questions can be either speculative questions or more technical questions. You may also want to write about the nature of the class meetings and group discussions: what works for you and what doesn't work, etc.
You are encouraged to cooperate with each other in working on anything in the course, but what you put in your journal should be you. If it is something that has emerged from work with other people, write down who you have worked with. Ideas that come from other people should be given proper attribution. If you have referred to sources other than the texts for the course, cite them.
Exposition is important. If you are presenting the answer to a question, explain what the question is. If you are giving an argument, explain what the point is before you launch into it. What you should aim for is something that could communicate to a friend or a colleague a coherent idea of what you have been thinking and doing in the course.
Your journal should be kept on loose leaf paper. Journals will be collected periodically to be read and commented on. If they are on loose leaf paper, you can hand in those parts which have not yet been read, and continue to work on further entries. Pages should be numbered consecutively and except when otherwise instructed, you should hand in only those pages which have not previously been read. Write your name on each page, and, in the upper right hand corner of the first page you hand in each time, list the pages you have handed in (e.g. [7,12] on page 7 will indicate that you have handed in 6 pages numbered seven to twelve).
Journals will be collected and read roughly every two weeks, the due dates are tentatively as follows:
10/4, 10/18,11/1, 11/15, 11/29, all on Wednesdays.
Homework
To supplement the discussion in class and assignments to be written about in your journals, we will assign readings from FPPA, together with accompanying homework. When you write the solutions to these homework problems, you should keep them separate from your journals.
Final project
At the end of the quarter, we will have a major project. The major project may be a paper investigating more deeply some topic we touch on lightly in class, or a topic that might arise in one of your other classes. Alternatively, you could design and carry out your own study. Or you might choose to do a computer-based project. To give you some ideas, a list of possible projects will be circulated. However, you are also encouraged to come up with your own ideas for projects.
Chance Fair
At the end of the course we will hold a CHANCE Fair where you will have a chance to present your project to the class as a whole, and to demonstrate your mastery of applied probability by playing various games of chance.
Resources
Materials related to the course will be kept on the public file server in a folder called Chance in the Math 5 folder. In particular this course description and the class assignments can be obtained there. In addition we will be regularly using the Chance database on the WWW. You can access this WWW site either from the Dartmouth Mathematics Department homepage or directly at
URL
http://www.geom.umn.edu/docs/snell/chance/welcome.html.
Library Reserve
Previous issues Chance magazine can be found on reserve in Kresge Library. Other materials that we will want to put on reserve will be in Baker Library.
Grades
Your grade in the course will be determined by your work on journal and class discussion, homework , project and (maybe) the final.
CLASS 1 PERCENTAGES
PERCENTAGES
Percents can be tricky. Here are some problems that point out some of the
pitfalls of percents.
DISCUSSION
1. Which would be better: to become 50% richer and then 50% poorer or to
become 50% poorer and then 50% richer, or to have your fortune remain
constant?
2. The recipe for pizza in Laurel's Kitchen says: Let the dough rise only
once, about 1 and 1/2 hours'. How long should you let the dough rise if you
use Fleischmann's Rapid Rise Yeast, whose package states that it `rises
50%faster'?
3. The following question was submitted to Marilyn vos Savant.
I'm having a problem with the illustration below, which was
captioned, ``Men consume about 76%of all alcoholic beverages.
Percentage consumed:"
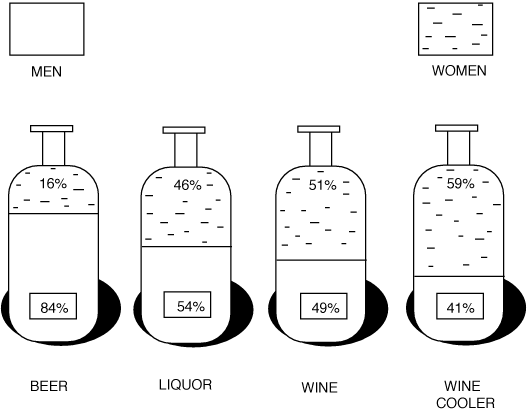
I understood the statement to mean: Of people who drink, 76%are
men, and 24%are women. But the illustration seems to suggest: Of
alcohol consumed, 57%is men, and 43%is by women (averaging the
four categories). Help! -K.B., Highland, Ind.
How should Marilyn answer this question?
4. A series of 12 monthly Tantalene injections ($450apiece) reduces the
5-year death toll from lemon meringue disease from 3 per 10,000,000 to 1 per
10,000,000. Suppose that you work for an advertising agency representing the
makers of Tantalene, and your job is to get doctors to prescribe Tantalene
injections for as many of their patients as possible. How would you express
this change in mortality rate using percents?
Homework assignments
Read Chapter 1 &2 and do the following problems. Page 19, No. 2, 3, 5, 6, 8,
12; Page 22, No. 2, 4, 9, 10, 11
Journal assignment for Wednesday, October 4
Read the current Chance News. Find an article that interests you and read
the full text of the article. Write your comments on the article in your
journal.
Homework assignment to be handed in with Journal due October 4.
CLASS 2
Activities
1. Accessing the Chance Database.
2. Stock picking: darts vs. you.
3. Class survey.
Discussions: Causation vs. Association
1. Simpson's paradox
There are two hospitals Mercy and Hope in your town. Under the new
national health plan you must choose one of these for health care. You
decide to base your decision on the success of their surgical teams.
Fortunately, under the new health plan the hospitals are required to
give data on the success of their operations broken down into five
broad categories of operations. You get the data for the two hospitals
and you find
You notice that, in all types of operations, Mercy hospital has a
higher success rate than Hope hospital and yet Hope has the highest
overall success rate. Which hospital would you choose and why?
2. Smoking and lung cancer
Watch and discuss the video.
CLASS 3
DISCUSSION
1. AIDS and AZT
Read the article "Study Challenges AZT Role As the Chief Drug for H.I.V."
- If drug A is better than drug B, and drug B is better than
nothing, is drug A better than nothing? Why or why not?
- Are you concerned about the dropout rate in this study? give your
reasons.
- What other issues or concerns do you have about this study?
2. Coin tosses
- In a study on how people perceive probability, Kahneman and
Tversky asked subjects ``which of the following two sequences is
more likely: H T H T T H or H H H T T T ? " Most people will say
the first sequence. Why do you think they say this?
- If a fair coin is tossed three times there will be 0, 1, 2, or 3
heads. How likely is each of these four possibilities?
Journal Assignment
- Read the articles in Chance database related to AIDS tests, and record
your comments.
- Perform experiments with coin tosses, do it in three ways: flip the>
coin, balance the coin on edge and strike the table, spin the coin on
edge. Do each trial 100 times, and record your probability.
CLASS 4: Probability
- Coin Tosses
- In a study on how people perceive probability, Kahneman and
Tversky asked subjects ``which of the following two sequences is
more likely: H T H T T H or H H H T T T ? " Most people will say
the first sequence. Why do you think they say this?
- If a fair coin is tossed three times there will be 0, 1, 2, or 3>
heads. How likely is each of these four possibilities?
- Beyond a reasonable doubt
A judge's charge to a jury might by like the following issued by Judge
Weinstein:
``If you entertain a reasonable doubt as to any fact or element
necessary to constitute the defendant's guilt, it is your duty to give
him the benefit of that doubt and return a verdict of not guilty. Even
where the evidence demonstrates a probability of guilt, if it does not
establish such guilt beyond a reasonable doubt, you must acquit the
accused. This doubt, however, must be a reasonable one; that is one
that is founded upon a real tangible substantial basis and not upon
mere caprice and conjecture. It must be such doubt as would give rise
to a grave uncertainty, raised in your mind by reasons of the
unsatisfactory character of the evidence or lack thereof. A reasonable
doubt is not a mere possible doubt. It is an actual substantial doubt.
It is a doubt that a reasonable man can seriously entertain. What is
required is not an absolute or mathematical certainty, but a moral
certainty." State v. Cage, 554 So.2d 39, 41 (La. 1989)
Discussion
- Do you feel a moral certainty that if you roll three dice, you
will not roll three 6's?
- Does it make sense to assign an actual probability to the notion
of a reasonable doubt? If so, what probability would you assign?
- Do you suppose that juries are ever really told what probability
to associate to the phrase `reasonable doubt'?
- What percent of the people on death row do you think are
innocent?
- In an article about the trial of John Bertsch and Jeffrey Hronis
accused of a 1985 kidnap, rape and murder case we read:
``The FBI's DNA tests in 1989 showed that the chances of a match
were 1 in 12 million for Bertsch and 1 in 8 million for Hronis. In
a re-testing in 1992 the FBI came up with 1 in 16,000 for Hronis>
and 1 in 200 for Bertsch."
Would the 1989 tests have satisfied ``beyond a reasonable doubt"?
What about the 1992 tests?
Journal Assignment
- Read Chapter 13, 14, 15 in the textbook. Do the following
problems to be handed in with your journal on Oct. 4th: P221,
#3,4,6,7,11; P235, #1,2,8,9,10; P243, #5,6,7.
- If you haven't done so, Perform experiments with coin tosses, do
it in three ways: flip a coin, balance a coin on edge and strike>
the table, spin a coin on edge. Do each trial 100 times, and
record the number of heads you get.
CLASS 7
Average class size
One of your friends at Harvard complains that the average class size at
Harvard is too big. He bets that your average class size is much smaller.
You decide to see if he is correct. Unfortunately, you are not sure how to
measure average class size.
Your first idea is to see if the registrar will give you a list of all the
classes this term and the size of each classes. Then you will just average
these to get average-class-size-1.
But then you have another idea. ``I'm a rather typical student and taking
three courses. Why don't I just take the average of the sizes of my
courses?" Then you worry that perhaps you are not completely typical so you
decide to ask a bunch of students to do this and take the average of their
responses. Better yet, you ask the registrar to do this for every student
and give you the average of all student averages. That surely should be what
your friend would mean by the average class size. You call this
average-class-size-2.
- Would these two averages be the same? If not which will be bigger? Which
would be more appropriate (a) for the President talking to Alumni (b) for
the chairman of the mathematics department arguing for more appointments in>
the math department?
- You could have asked about the median class size instead of the mean.
With either of these methods do you think that the median would be smaller
or larger than the mean?
- Make an estimate for both of these averages just using class size
information from members of your group.
- Do you have any other method to suggest for calculating average class
size?
Homworks
Read Chapters 3,4 , and 5 of the text. Do the following exercises: Review
Exercises in Chapter 4: page 70: #2, 4, 8, 9, 11, 12 Review Exercises in
Chapter 5: page 87: #4, 7, 8, 9,12
CLASS 8
Read the two articles: "He's got their number" and "Physicists makes sense of market with bobbing corks, not chaos". Answer following questions:
(1) Which article do you think is better written from the point of wiew of really explaining what is going on?
(2) What more would you need to know to really understand what is going on?
CLASS 9
- Read the article "Women give weight study a pounding", and discuss the following
questions:
- What did Susan Hankinson mean by "the mathematical techniques the
researchers were compelled to use made it necessary to round off the risk factor
to 20 percent"?
- The article states that "the risk that a nonsmoking young or middle-aged
woman will die in a given year is so small that a 20 percent rise verges on the
imponderable. The jump in odds translates roughly to a rise from 10 in 10,000 to
12 in 10,000." Is this a good way to explain the risk of being 20 percent over the
recommended weight? Do you think they mean any 20 percent increase in
weight?
- Read the article "Study finds a soaring rate of obesity in U.S. Children",
discuss the question: Is this a good way to measure obesity?
Assignments
Read the current Chance news, find some articles you are interested in and record
your opinions in your journal.
CLASS 10 The Monte Hall Problem
The Monte Hall Problem
Here is the infamous Monte Hall problem, as it appeared in the Parade
Magazine of 9 September 1990:
Suppose you're on a game show, and you're given the choice
of three doors: Behind one door is a car; behind the others,
goats. You pick a door, say number 1, and the host, who
knows what's behind the doors, opens another door, say
number 3, which has a goat. He then says to you, ``Do you
want to pick door number 2?'' Is it to your advantage to
switch your choice?
Discussion
- Should you stick or switch?
- Design and carry out experiments to check your conclusion.
- What assumptions does your answer depend on?
- Discuss other plausible assumptions, and how they would affect
your answer.
Class 12
Assignments
Read Chapters 8,9,10 in the textbook, do the following exercises:
P127, #1,2,9; P145, #3,7,9; P167, #1,3,8,9.
Class 13 Coke vs. Pepsi: Designing an experiment
Handout
The article "Linking technology and health groups to find best care" from the current Chance News
Coke vs. Pepsi: Designing an experiment
Identify a member of your group who claims to be able to tell the difference between Pepsi and Coke. (Coke Classic, that is; accept no substitutes!) Design and carry out an experiment to test whether this is true. Remember that one swallow doth not a summer make: Don't certify your taste-tester just on the basis of one experiment. Decide exactly what data you will collect and what you will do with the data before you start collecting it. Also, remember the story of clever Hans, and consider the benefits of a double�blind study.
Journal Assignments
- Read the article "Linking technology and health groups to find best care". Consider such questions as: Is this an effective method to find the best care? What do you think of this use of medical records? Record your thoughts in your journal.
- We would like you to include in your journal on November 1 a brief description of your proposed final project.
Class 14
Discussion
Read the article "Probability experts may decide Pennsylvania vote"
and discuss the following questions:
- How did Professor Ashenfelter get that Republican's 564 vote edge on
the machines should have led to a 133-vote advatange in absentee ballots?
- The Times says that "The probability that the unusual results ...were
simply caused by random variations in voting patterns is just 6%." Comment.
- Do you think that large-scale fraud took place during this election? If so,
who do you think would have won the election if there had been no fraud?
- What would you do if your were the judge? Seat Marks? Call a new
election? Seat Stinson?
Class 15 Correlation: Taste and price for cookies
Correlation: Taste and price for cookies
- Divide into groups to design an experiment to rank cookies: again
you probably want to consider the issues raised in Chapters 1 & 2.
- Taste cookies and rank them.
| Cookies |
C1 |
C2 |
C3 |
C4 |
C5 |
C6 |
C7 |
C8 |
C9 |
C10 |
| Rank |
|
|
|
|
|
|
|
|
|
|
Journal
We will make the result of the experiment available on Public. Analyze the
data and record your findings in your next journal.
Class 16 Guest speaker: Nancy Surrel
Guest speaker: Nancy Surrel
Nancy writes press releases for Dartmouth College. She was a science writer for
the Valley News before she came to Dartmouth. The topic of her talk is on reporting scientific news, especially issues related to the NewYork Times article " " (which was
discussed in Class ??). Some questions asked by the students were: " If you know that
certain scientific results such as a cup of wine a day is good for the heart is very preliminary, why do you keep writing about them?", " What are the rules governing protecting your sources?", "What is your best work?". Issues that were discussed included: the role of news in guiding your behavior, the power of news, scientific
study and funding, objective reporting, etc.
Class 17 Experiment: Sampling
Discussion: Polls
Guest speakers: we will have two Dartmouth students who are doing polls to
tell us about their experience.
Experiment: Sampling
We will try to decide the percentage of black and white beans in a mixed bag. Issues
to consider: how large is your sample? how representative is your sample?
how big is the possible error?
Homeworks & Journal
- Read Chapters 19-23. Do the following exercises: P324, #6,7. P340, #2,3,6,9,10.
P357, #1,3,8,11. P389, #1,2.
- Read the current issue of Chance News, find an article and report your thoughts in your journal.
Quebec referendum
Find out what you can about the Quebec referendum, e.g., the poll over the weekend and the vote today. We will discuss this on Wednesday.
Class 18 Standard Error: Errors in sampling
Discussion: Polls
- The results of the WMUR-TV poll we discussed last time.
- Read the 1996 presidential poll, what do you think about the questions?
- NewYork Times article (10/26/95): "Americans reject big medicare cuts, a new poll finds".
Read the part in the box "How the poll was conducted". It says that the error is
3%, how do they get that?
Standard Error: Errors in sampling
- An introduction to standard error
-
Discuss the Bean-counting experiment.
| groups |
# of beans in the sample |
# of White beans in the sample |
% of white beans in the sample |
Margin of error |
95% Confidence interval |
| 1 |
430 |
253 |
58.84 |
4.75 |
(54.09,63.58) |
| 2 |
689 |
347 |
50.36 |
3.81 |
(46.55,54.17) |
| 3 |
242 |
137 |
56.61 |
6.37 |
(50.24,62.98) |
| 4 |
739 |
399 |
53.99 |
3.67 |
(50.33,57.66) |
| 5 |
724 |
432 |
59.67 |
3.65 |
(56.02,63.31) |
| 6 |
1804 |
1005 |
55.71 |
2.34 |
(53.37,58.05) |
| 7 |
1063 |
533 |
50.14 |
3.07 |
(47.07,53.21) |
| 8 |
934 |
521 |
55.78 |
3.25 |
(52.53,59.03) |
| 9 |
1027 |
698 |
67.96 |
2.91 |
(65.05,70.88) |
| 10 |
827 |
468 |
56.59 |
3.45 |
(53.14,60.04) |
| 11 |
2403 |
1372 |
57.10 |
2.02 |
(55.08,59.11) |
| total |
11062 |
6165 |
55.73 |
0.95 |
(54.79,56.68) |
Class 21 Tests for significance
Discussion: Tests for significance
- Someone gives you a coin and claims that it is a fair coin.
Do you believe the claim if
- you toss it 100 times and get 55 heads?
- you toss it 100 times and get 75 heads?
- you toss it 10000 times and get 4000 heads?
- In our class survey, the mean of the 35 male students' SAT Verbal score is
640, with standard deviation 74. For the 24 female students, the mean is 700 and the
standard deviation is 46. We want to find out whether this difference can reasonably
be explained by chance variation?
- If the differences are all due to chance, what do we expect the difference to be?
- How much spread do the differences have? (standard deviation)
- How might we estimate the probability of such a difference occuring by chance?
- In our class survey about smoking habits, of the 26 female students, 18
don't smoke, 8 smoke occasionally and non smoke regularly. Of the 35 male students, 25 don't smoke,
5 smoke occasionally and 5 smoke regularly. We want to find out whether the smoking
habits are independent of gender?
- If they are really independent, what do we expect the numbers to be?
- How might we measure the difference between our data and the expected data?
- How might we estimate the probability of such a difference occuring by chance?
Homework
Read Chapters 26-29, do the following exercises:P449, #2,4,5; P469,#1,2; P490, #1,2,5.
Class 22 Tests for significance
Discussion: Mortality and alcohol
- Video: The "60 Minutes" report.
- Divide into groups to discuss the following questions related to the article "Mortality associated with moderate intakes of wine, beer, or spirited
- What is meant by the "Unshaped relation"? How did the research explain that?
- Why did the research worry about possible confounding factors? which factors do you think are maybe confounding? Why is the study so large? why didn't they just study 1000 people? What do you think about the quality of the research'? Will this research change your life, and why?
Class 24 Coincidences, coincidences, ...
- Write down an "amazing coincidence" that you have personally experienced.
- Valley News article "Bible's word patterns suggest divine writing" (11/3/95).
- The birthday problem.
- New York Times article on USAir's safety records (9/11/94)
