NOTE: Problems have been designed so that they may use information given in other problems, but each problem can be worked without your having worked the others.
The problems or their parts are assigned points in the margin, totaling 115. You may select any collection totaling 100 points. (When a problem has several parts, you may select whichever parts you wish.) Write the numbers of the problems (and parts) on which you wish to be graded, on the cover of your exam book.
The examination is in the form of a story. It is suggested that you read it once quickly before selecting the problems you decide to work.
Only the names have been changed to protect the innocent.
Once upon a time there was a rumble at the bottom of the ocean. With probability 1/3, one volcano would arise, while with probability 2/3 two volcanos would arise. Given that one arises, there is probability 3/4 that enough lava spouts up to make an island (and probability 1/4 that no island forms). But, if two volcanos arise, the lava is split between them, and the conditional probability that i islands form is ( 3 - i ) / 6 for i = 0, 1, 2.
5 (a) Determine whether or not the event that a single volcano forms is independent of the event that a single island forms.
10 (b) Find the probability function of the rv N = number of islands formed.

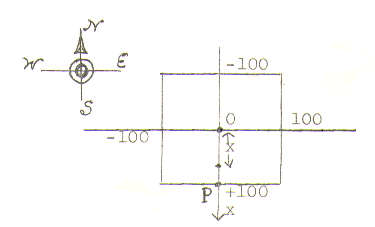
Actually, a single island formed. Its shape was a square with sides parallel to the compass directions, 200 meters on a side.
Over the years, simple plants washed ashore and grew. The barren rock became soil. A lovely and edible flower eventually covered much of the island.
The island had a peak at its center (0 in diagram), and the slope was such that little sun hit the north half of the island, while the noonday temperature (oc) of the soil a distance x meters south of the center was 20 + x2 / 500 for 0 < = x < = 100.
A pair of insects land at random (uniform distribution) on the segment OP between the center and the point on the shore due south of it, and their eggs are deposited there.
10 (a) Show that the probability density function of the noonday temperature T where they land is
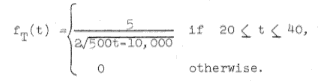
[Hint: do NOT use (a)!]
5 (c) The eggs only hatch if they land where the noonday temperature is between 25 and 27.2. What is the probability that this happens?
One night a great storm washes a cherry ashore. The cherry lands exactly at the center O of the island, and its seed produces a tree. The number of full years Y that such a tree lives has a geometric law,
Here p can depend on various environmental factors, as we shall see later.
A tree can only bear fruit and thus have progeny if it lives at least 10 years.
How large must p be in order that the tree has a 50-50 chance od bearing fruit?
The insect eggs of problem 2 hatch, and generations of insects follow.
A man is shipwrecked on the island.
No food floats ashore with him, but he can survive on the lovely and edible flower mentioned earlier.
What does float ashore with him is a crate of MIRA-KILL, the greatest insect-destroying marvel of modern technology.
The man is a great scientist.
He notices that the insects have started to devour the leaves of the cherry tree, which would drastically reduce the quantity p mentioned in problem 3.
This year's insects die, but their eggs linger on.
In order to make sure p is large enough to give the tree the 50-50 chance mentioned in problem 3, the scientist will spread his MIRA-KILL over the island so that it has a good chance of destroying the insect eggs and saving the tree. If he can destroy all the eggs except perhaps one, this insect species will die out.
There are 106 eggs on the island, and MIRA-KILL is so potent that each egg has only a chance of 2 x 10-6 of surviving, with survival being independent from egg to egg.
What (approximately) is the chance that at most one egg survives?
The scientist once took a probability course.
To relieve his boredom on the island, he tries to recall and, apply some of the ideas he learned.
He notices that the actual amount of MIRA-KILL which lands on
each egg, in milligrams, is uniformly distributed between 0 and
4, and the amounts on different eggs are independently
distributed.
(Perhaps you realize that a chemical balance also floated
ashore!)
10 (a) He shows that the mean and standard deviation of the amount of MIRA-KILL on a random egg are 2 and 2/(squareroot of 3) respectively. Do the same.
10 (b) He notices a pair of eggs next to each other, and wonders about the total amount Z of poison on the two eggs. He remembers something called the MGF of Z, and computes that it is
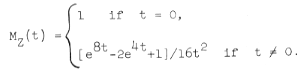
10 (c) He suspects that the total amount of poison in the two eggs is likely to be between 2 and 6 milligrams. But, having computed Mz, he finds he doesn't know how to use it to find the probability of this event 2 < = z < = 6. Compute this probability without making use of Mz.
5 (d) He remembers, from his probability course, that he can use the result of (a) to show that EZ = 4. But he doubts whether he could have reached that conclusion if he knew only that the amount of MIRA-KILL on each egg had marginal distribution as given above, but that the amounts on different eggs might not be independent. Explain briefly whether he is right to have such doubts.
Enough insect eggs are killed (as described in problem 4) that p is large enough, and the tree is lucky enough, that it lives exactly 10 years, so it produces one crop of fruit.
The seeds would ordinarily be spread by a migrating flock of birds which pass through the island.
Unfortunately, the poisoned insect eggs are eaten by the birds before the cherries are ripe. Each bird eats 75 eggs, and dies if it gets more than 120 milligrams of MIRA-KILL.
5 (a) Use the result of Problem 5(a) to find the mean and variance of the total amount of poison a bird eats.
10 (b) Use the result of (a) to show that the probability that a given bird survives is approximately
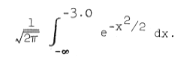
All the birds die.
Moreover, they never had a chance to eat the cherries, which therefore drop directly under the tree, where by now there are too many leaves for the seeds to reach the soil and germinate.
The tree dies, childless.
The lovely and edible flowers, which had relied on the insects for pollenization, gradually die out now that the insects are gone.
The man has nothing to eat and gradually starves. He finally manages to catch a small fish. If only he had 4 fish, he could survive for a week. He notices that these fish attract each other, and hits upon a scheme for catching more:
He holds his fish in the water. With probability 1/2 another fish will swim near slowly enough that the man can capture it with other hand. But the other possibility of probability 1/2 is that this other fish will dart at his hand very rapidly and bite it, and he will lose his first fish, too, and have nothing. However, if he gets a second fish in this way, he can use one of his two fish to try to attract a third fish (or lose the second one) in the same way, and so on. He stops when his total gets to O or 4 fish, whichever happens sooner.
What is the expected number of fish he ends up with? (A one line explanation should accompany your answer.)
(FOR ENTERTAINMENT ONLY, O POINTS; WORK ONLY IF YOU HAVE TIME, BUT PLEASE DO SO IF YOU HAVE IT, TO SATISFY THE INSTRUCTOR'S AND TAPS CURIOSITY!)
The fish escaped.
The man died of hunger.
The island, devoid of vegetation, was eroded by the rain and the sea, until it disappeared.
Many ages later, the ocean floor rumbles again. A single square island, 200 meters on side, arises, its center uniformly distributed throughout the ocean.
(a) Is it correct that the probability is 0 that the second island's center appears exactly where the first island's center was?
(b) If your answer to (a) is that this is indeed an event of probability 0, does this mean that it's impossible for history exactly to repeat itself?
MORAL: Performing well in a probability course may not be as earth shaking a consideration as it seems to you at this moment ...